Integrate cos^32x

To integrate cos^32x, also written as ∫cos³2x dx, cos cubed 2x, cos^3(2x), and (cos 2x)^3, we start by using a standard trig identity to change the form.

It is much simpler if you do it this way... If you bring out one of the cos2x terms, then you get an expression with a cos squared term.

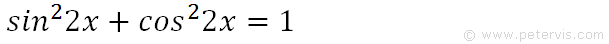
We recall the Pythagorean trig identity and double the angles throught.

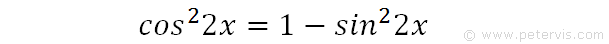
We rearrange the trig identity for the cos2x squared term.

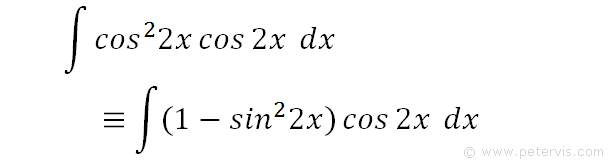
We substitute the trig identity into our integration problem.

We let u = sin2x.

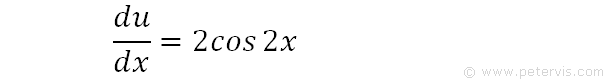
Then du/dx = 2cos2x. If you want to know how to do this differentiation, then I have shown this at the bottom of the page.

We rearrange the previously found expression for du. As you can see, the cos2x dx part is just like the part in our integration problem. The only issue we have is with the constant 2, otherwise we would be able to substitute all of it with du.

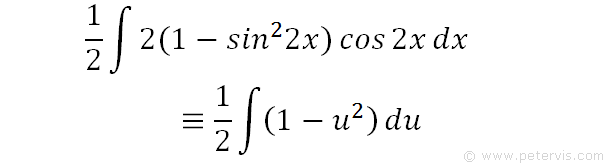
If we multiply ½ by 2, then the result is 1 and we have not changed anything. Therefore play this little trick to change the form, so that we can substitute du into the expression. Hence, with the change of form, we can replace 2cos2x dx with du. We also replace the sin2x term inside the brackets with u, to give a new expression that means the same thing.

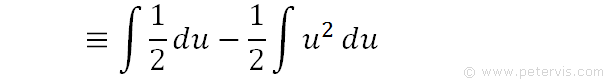
We open the brackets and multiply out the ½. We also integrate each term separately. As you can see, it means the same thing as before.

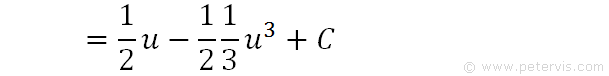
We then integrate u w.r.t. du. This is the easy part.

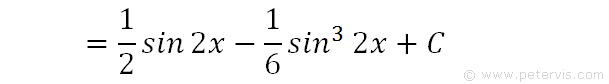
We then replace u with sin2x to give the final answer.

Differentiate sin2x