The Quadratic Equation

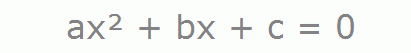
The quadratic equation formula describes the general shape of a parabola, and the coefficients a, b, and c determine its size and position.

Formula

The formula to solve a quadratic equation is very simple. Almost all high-school students should know this.

Discriminant

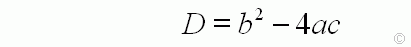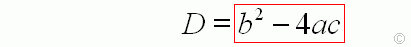
The discriminant part indicates whether the solutions of x will be real or imaginary.

Discriminant > 0

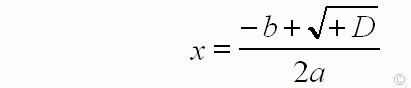
When the discriminant is a positive number, there are two real roots to the equation, given by the formulas shown above.

Discriminant = 0

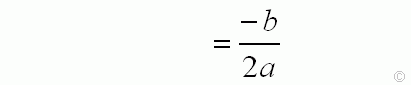
When the discriminant is equal to zero, there is only one real solution.

Discriminant < 0

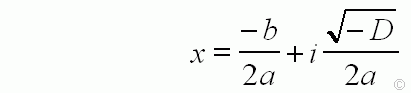
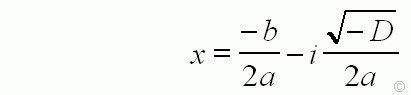
When the discriminant is negative, there are no real roots, and two imaginary roots, as shown above. The roots form a complex conjugate pair.

Quiz

Here is a very simple pub type question and answer, ideal for the so-called "educated" educators. Very few in the UK can answer this one correctly.

Question

Who was the first person to give an explicit solution for a quadratic equation with negative numbers?

Answer

Brahmagupta

Question and Answer

Here are some real-life example questions and answers. Consider these as online worksheets. It might prove useful to GCSE students and teachers.

This Article Continues...